Distribution of Chosen Lottery Numbers

In this example we saw that, in the first 1172 weeks of the lottery the number 38 was chosen 175 times whereas the number  41 was chosen only 115 times. Is there something special about these numbers?  

In fact the apparently strange distribution for the number of times each number is chosen is entirely predictable, as we can now show (you can see this in action by running this model in AgenaRisk).

Each of the 49 numbers is equally likely to be selected. Since six numbers are chosen each week, for each number there is a 6/49 probability that it will be chosen. For any number of weeks the number of times a given number will be chosen is a binomial distribution where the number of 'trials' is simply the number of weeks and the 'probability of success' is 6/49.  If we run this binomial distribution in AgenaRisk and enter the number of trials as 1172 we get the following distribution for the number of times a given number will be chosen:




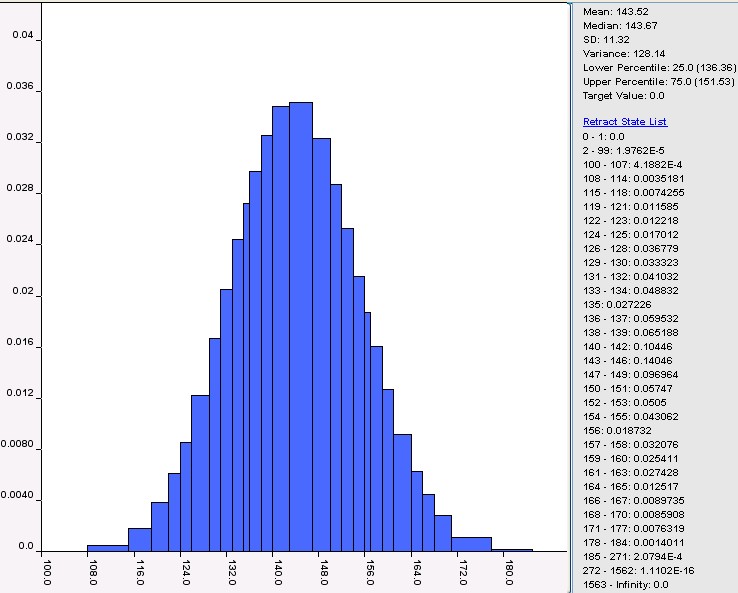
For example, looking at the percentile information shown you can see that there is a 0.5 probability that the number of times the number will be chosen is outside the range 136-152. In other words, since there are 49 numbers we can actually expect about half of these (24) to be chosen either less than 136 times or more than 152 times. That indeed is roughly what happened in the real sample. Similarly, the probability that the number will be chosen more than 171 times is about 0.012. Since there are 49 numbers, the probability that at least one of them is chosen more than 171 times is quite high (about 0.45). Hence the fact that one number was chosen 175 times is not unusual.